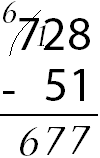Difference between revisions of "Arithmetic" - New World Encyclopedia
| Line 1: | Line 1: | ||
| − | '''Arithmetic''' or '''arithmetics''' (from the [[Greek language|Greek]] word ''αριθμός'', meaning "number") is the oldest and most elementary branch of mathematics, used by almost everyone, for tasks ranging from simple daily counting to advanced [[science]] and [[business]] calculations. In common usage, the word refers to a branch of (or the forerunner of) [[mathematics]] which records elementary properties of certain ''operations'' on [[number]]s. Professional [[mathematician]]s sometimes use the term ''higher arithmetic''<ref>Davenport, Harold (1999). ''The Higher Arithmetic: An Introduction to the Theory of Numbers (7th ed.).'' Cambridge, England: Cambridge University Press. ISBN 0-521-63446-6.</ref> as a synonym for [[number theory]], but this should not be confused with [[elementary arithmetic]]. | + | '''Arithmetic''' or '''arithmetics''' (from the [[Greek language|Greek]] word ''αριθμός'', meaning "number") is the oldest and most elementary branch of mathematics, used by almost everyone, for tasks ranging from simple daily counting to advanced [[science]] and [[business]] calculations. In common usage, the word refers to a branch of (or the forerunner of) [[mathematics]] which records elementary properties of certain ''operations'' on [[number]]s. Professional [[mathematician]]s sometimes use the term ''higher arithmetic''<ref>Davenport, Harold (1999). ''The Higher Arithmetic: An Introduction to the Theory of Numbers (7th ed.).'' Cambridge, England: Cambridge University Press. ISBN 0-521-63446-6.</ref> as a synonym for [[number theory]], but this should not be confused with [[elementary arithmetic]]*. |
| + | |||
| + | The traditional arithmetic operations are [[addition]]*, [[subtraction]]*, [[multiplication]]*, and [[division (mathematics)|division]]*, although more advanced operations (such as manipulations of [[percentage]]s, [[square root]], [[exponentiation]], and [[logarithm|logarithmic functions]]) are also sometimes included in this subject. Any set of objects upon which all four operations of arithmetic can be performed (except [[division by zero]]*), and wherein these four operations obey the usual laws, is called a ''[[Field mathematics|field]]*''. | ||
==History== | ==History== | ||
| − | + | Our knowledge of the prehistory of arithmetic is limited by a small number of artifacts indicating a clear conception of addition and subtraction, the best-known being the [[Ishango Bone]]* from [[Africa]], dating from 18,000 B.C.E. | |
It is clear that the [[Babylonian]]s had solid knowledge of almost all aspects of elementary arithmetic circa 1850 B.C.E., historians can only infer the methods utilized to generate the arithmetical results (see [[Plimpton 322]]). Likewise, a definitive [[algorithm]] for multiplication and the use of [[unit fraction]]s can be found in the [[Rhind Mathematical Papyrus]] dating from Ancient Egypt circa 1650 B.C.E. | It is clear that the [[Babylonian]]s had solid knowledge of almost all aspects of elementary arithmetic circa 1850 B.C.E., historians can only infer the methods utilized to generate the arithmetical results (see [[Plimpton 322]]). Likewise, a definitive [[algorithm]] for multiplication and the use of [[unit fraction]]s can be found in the [[Rhind Mathematical Papyrus]] dating from Ancient Egypt circa 1650 B.C.E. | ||
| − | In the [[Pythagoreanism|Pythagorean school]], in the second half of the | + | In the [[Pythagoreanism|Pythagorean school]], in the second half of the sixth century B.C.E., arithmetic was considered one of the four quantitative or mathematical sciences (''Mathemata''). These were carried over in mediæval universities as the ''[[Quadrivium]]*'' which, together with the ''[[Trivium]]*'' of grammar, rhetoric and dialectic, constituted the ''septem liberales artes'' (seven liberal arts). |
| − | Modern algorithms for arithmetic (both for hand and electronic computation) were made possible by the introduction of [[Arabic numerals]] and [[decimal]] place notation for numbers. Although it is now considered elementary, its simplicity is the culmination of thousands of years of mathematical development. By contrast, the ancient mathematician [[Archimedes]] devoted an entire work, [[The Sand Reckoner]], to devising a notation for a certain large integer. The flourishing of algebra in the medieval Islamic world and in Renaissance Europe was an outgrowth of the enormous simplification of computation through decimal notation. | + | Modern algorithms for arithmetic (both for hand and electronic computation) were made possible by the introduction of [[Arabic numerals]] and [[decimal]] place notation for numbers. Although it is now considered elementary, its simplicity is the culmination of thousands of years of mathematical development. By contrast, the ancient mathematician [[Archimedes]] devoted an entire work, [[The Sand Reckoner]]*, to devising a notation for a certain large integer. The flourishing of algebra in the medieval Islamic world and in Renaissance Europe was an outgrowth of the enormous simplification of computation through decimal notation. |
==Decimal arithmetic== | ==Decimal arithmetic== | ||
| Line 17: | Line 19: | ||
[[Algorism]] comprises all of the rules of performing arithmetic computations using a decimal system for representing numbers in which numbers written using ten symbols having the values 0 through 9 are combined using a place-value system (positional notation), where each symbol has ten times the weight of the one to its right. | [[Algorism]] comprises all of the rules of performing arithmetic computations using a decimal system for representing numbers in which numbers written using ten symbols having the values 0 through 9 are combined using a place-value system (positional notation), where each symbol has ten times the weight of the one to its right. | ||
This notation allows the addition of arbitrary numbers by adding the digits in each place, which is accomplished with a 10 x 10 addition table. (A sum of digits which exceeds 9 must have its 10-digit carried to the next place leftward.) One can make a similar algorithm for multiplying arbitrary numbers because the set of denominations {...,10<sup>2</sup>,10,1,10<sup>-1</sup>,...} is closed under multiplication. Subtraction and division are achieved by similar, though more complicated algorithms. | This notation allows the addition of arbitrary numbers by adding the digits in each place, which is accomplished with a 10 x 10 addition table. (A sum of digits which exceeds 9 must have its 10-digit carried to the next place leftward.) One can make a similar algorithm for multiplying arbitrary numbers because the set of denominations {...,10<sup>2</sup>,10,1,10<sup>-1</sup>,...} is closed under multiplication. Subtraction and division are achieved by similar, though more complicated algorithms. | ||
| − | |||
| − | |||
| − | |||
==Addition (+)== | ==Addition (+)== | ||
{{main|Addition}} | {{main|Addition}} | ||
| − | + | [[Image:Addition01.svg|right|thumb|120px|An example of addition: 3 + 2 = 5, using apples, a popular choice in textbooks.<ref>From Enderton (p.138): "...select two sets ''K'' and ''L'' with card ''K'' = 2 and card ''L'' = 3. Sets of fingers are handy; sets of apples are preferred by textbooks."</ref>]] | |
| − | + | Addition is the basic [[Operator|operation]]* of arithmetic. In its simplest form, addition combines two [[number]]s, the ''addends'' or ''[[term (mathematics)|terms]]*'', into a single number, the ''sum''. | |
| − | + | The result of adding two quantities ''a'' and ''b'' is their ''sum'', ''a'' + ''b''. It is sometimes phrased as "''a'' more than ''b''," or "''b'' more than ''a''." For example, 3 + 2 = 5, since 5 is 2 more than 3. | |
| − | + | Adding more than two numbers can be viewed as repeated addition; this procedure is known as [[summation]] and includes ways to add infinitely many numbers in an [[series (mathematics)|infinite series]]; repeated addition of the number [[1 (number)|one]] is the most basic form of [[counting]]. | |
| − | [[ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | Addition also models many related processes, including joining two collections of objects, repeated incrementation, moving a point across the number line, and representing two successive translations as one. | |
| − | |||
| − | |||
| − | |||
=== Notation and terminology === | === Notation and terminology === | ||
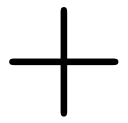
[[Image:PlusCM128.svg|right|128px]] | [[Image:PlusCM128.svg|right|128px]] | ||
| + | |||
Addition is written using the [[Plus and minus signs|plus sign]] "+" between the terms; that is, in [[infix notation]]. The result is expressed with an [[equals sign]]. For example, | Addition is written using the [[Plus and minus signs|plus sign]] "+" between the terms; that is, in [[infix notation]]. The result is expressed with an [[equals sign]]. For example, | ||
:1 + 1 = 2 | :1 + 1 = 2 | ||
| Line 60: | Line 52: | ||
This is to be distinguished from ''factors'', which are [[multiplication|multiplied]]. | This is to be distinguished from ''factors'', which are [[multiplication|multiplied]]. | ||
Some authors call the first addend the ''augend''. In fact, during the [[Renaissance]], many authors did not consider the first addend an "addend" at all. Today, due to the symmetry of addition, "augend" is rarely used, and both terms are generally called addends.<ref>Schwartzman p.19</ref> | Some authors call the first addend the ''augend''. In fact, during the [[Renaissance]], many authors did not consider the first addend an "addend" at all. Today, due to the symmetry of addition, "augend" is rarely used, and both terms are generally called addends.<ref>Schwartzman p.19</ref> | ||
| + | |||
| + | === Properties === | ||
| + | |||
| + | Addition is [[commutative]] and [[associative]] so the order in which the terms are added does not matter. <<Please define the terms "commutative" and "associative" here. You may consult articles by these names in Wikipedia.>> The [[identity element]] of addition (the [[additive identity]]) is 0, that is, adding zero to any number will yield that same number. Also, the [[inverse element]] of addition (the [[additive inverse]]) is the opposite of any number, that is, adding the opposite of any number to the number itself will yield the additive identity, 0. For example, the opposite of 7 is (-7), so 7 + (-7) = 0. | ||
==Subtraction (−)== | ==Subtraction (−)== | ||
{{main|Subtraction}} | {{main|Subtraction}} | ||
| − | + | [[Image:Subtraction01.svg|right|thumb|180px|An example of subtraction: "5 - 2 = 3".]] | |
| + | [[Image:Subtraction Example Image 1.PNG|right|thumb|180px|A subtraction problem.]] | ||
| − | Subtraction | + | Subtraction is essentially the opposite of addition. It is denoted by a [[plus and minus signs|minus sign]]* "−" in [[infix notation]]*. |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
The traditional names for the parts of the [[formula]] | The traditional names for the parts of the [[formula]] | ||
| Line 77: | Line 69: | ||
are ''minuend'' (''c'') − ''subtrahend'' (''b'') = ''difference'' (''a''). The words "minuend" and "subtrahend" are virtually absent from modern usage; Linderholm charges "This terminology is of no use whatsoever."<ref>Linderholm p.42</ref> However, "difference" is very common. | are ''minuend'' (''c'') − ''subtrahend'' (''b'') = ''difference'' (''a''). The words "minuend" and "subtrahend" are virtually absent from modern usage; Linderholm charges "This terminology is of no use whatsoever."<ref>Linderholm p.42</ref> However, "difference" is very common. | ||
| − | Subtraction is used to model several closely related processes: | + | If the minuend is larger than the subtrahend, the difference will be positive; if the minuend is smaller than the subtrahend, the difference will be negative; and if they are equal, the difference will be [[0 (number)|zero]]. |
| + | |||
| + | Subtraction is used to model several closely related processes. For example: | ||
#From a given collection, take away (subtract) a given number of objects. | #From a given collection, take away (subtract) a given number of objects. | ||
#Combine a given measurement with an opposite measurement, such as a movement right followed by a movement left, or a deposit and a withdrawal. | #Combine a given measurement with an opposite measurement, such as a movement right followed by a movement left, or a deposit and a withdrawal. | ||
#Compare two objects to find their difference. For example, the difference between $800 and $600 is $800 − $600 = $200. | #Compare two objects to find their difference. For example, the difference between $800 and $600 is $800 − $600 = $200. | ||
| − | In [[mathematics]], it is often useful to view or even define subtraction as a kind of | + | === Properties === |
| + | |||
| + | Subtraction is neither commutative nor associative. For that reason, it is often helpful to look at subtraction as addition of the minuend and the opposite of the subtrahend, that is ''a'' − ''b'' = ''a'' + (−''b''). When written as a sum, all the properties of addition hold. | ||
| + | |||
| + | In [[mathematics]], it is often useful to view or even define subtraction as a kind of addition, the addition of the opposite. We can view 7 − 3 = 4 as the sum of two [[term]]s: seven and negative three. This perspective allows us to apply to subtraction all of the familiar rules and nomenclature of addition. Although subtraction is not [[associative]] or [[commutative]], the addition of signed numbers is both. | ||
===Basic subtraction: integers=== | ===Basic subtraction: integers=== | ||
| Line 115: | Line 113: | ||
77777777777 | 77777777777 | ||
| + | |||
In [[mathematics]], '''multiplication''' is an elementary arithmetic [[operation (mathematics)|operation]]. When one of the numbers is a [[whole number]], '''multiplication''' is the repeated [[addition|sum]] of the other number. | In [[mathematics]], '''multiplication''' is an elementary arithmetic [[operation (mathematics)|operation]]. When one of the numbers is a [[whole number]], '''multiplication''' is the repeated [[addition|sum]] of the other number. | ||
| Line 126: | Line 125: | ||
===Computation=== | ===Computation=== | ||
| − | |||
The standard methods for multiplying numbers using pencil and paper require a [[multiplication table]] of memorized or consulted products of small numbers (typically any two numbers from 0 to 9), however one method, the [[peasant multiplication]] algorithm, does not. | The standard methods for multiplying numbers using pencil and paper require a [[multiplication table]] of memorized or consulted products of small numbers (typically any two numbers from 0 to 9), however one method, the [[peasant multiplication]] algorithm, does not. | ||
| − | Multiplying numbers to more than a couple of decimal places by hand is tedious and error prone. [[Common logarithm]]s were invented to simplify such calculations. The [[slide rule]] allowed numbers to be quickly multiplied to about three places of accuracy. Beginning in the early [[twentieth century]], mechanical [[calculator]]s | + | Multiplying numbers to more than a couple of decimal places by hand is tedious and error prone. [[Common logarithm]]s were invented to simplify such calculations. The [[slide rule]] allowed numbers to be quickly multiplied to about three places of accuracy. Beginning in the early [[twentieth century]], mechanical [[calculator]]s automated multiplication of up to 10 digit numbers. Modern electronic [[computer]]s and calculators have greatly reduced the need for multiplication by hand. |
===Terminology=== | ===Terminology=== | ||
| − | The two numbers being multiplied are formally called the | + | The two numbers being multiplied are formally called the ''multiplicand'' and the ''multiplier'', respectively. (Some write the multiplier first, and say that 7 × 4 stands for |
| − | ''multiplicand'' and the ''multiplier'', respectively. | + | 4 + 4 + 4 + 4 + 4 + 4 + 4, but this usage is less common.) The difference was important in [[Roman numerals]] and similar systems where multiplication is transformation of symbols and their addition. For example, to multiply VII by XV one changes the VII to LXX (multiplying VII by X) plus XXV (V times V) plus X (II times V), but to multiply XV by VII one changes XV into LXXV (XV times V) plus XV plus XV (each XV times I). |
| − | (Some write the multiplier first, and say that 7 × 4 stands for | ||
| − | 4 + 4 + 4 + 4 + 4 + 4 + 4, but this usage is less common.) | ||
| − | The difference was important in [[Roman numerals]] and similar systems where multiplication is transformation of symbols and their addition. For example, to multiply VII by XV one changes the VII to LXX (multiplying VII by X) plus XXV (V times V) plus X (II times V), but to multiply XV by VII one changes XV into LXXV (XV times V) plus XV plus XV (each XV times I). | ||
Because of the [[commutative property]] of multiplication, there is generally no need to distinguish between the two numbers so they are more commonly referred to as the ''factors''. The result of the multiplication is referred to as the ''product''. | Because of the [[commutative property]] of multiplication, there is generally no need to distinguish between the two numbers so they are more commonly referred to as the ''factors''. The result of the multiplication is referred to as the ''product''. | ||
| Line 160: | Line 155: | ||
If the terms are not written out individually, then the product may be written with an [[ellipsis]] to mark out the missing terms, as with other series operations (like sums). | If the terms are not written out individually, then the product may be written with an [[ellipsis]] to mark out the missing terms, as with other series operations (like sums). | ||
Thus, the product of all the natural numbers from 1 to 100 can be written <math>1 \cdot 2 \cdot \ldots \cdot 99 \cdot 100</math>. This can also be written with the ellipsis vertically placed in the middle of the line, as <math>1 \cdot 2 \cdot \cdots \cdot 99 \cdot 100</math>. | Thus, the product of all the natural numbers from 1 to 100 can be written <math>1 \cdot 2 \cdot \ldots \cdot 99 \cdot 100</math>. This can also be written with the ellipsis vertically placed in the middle of the line, as <math>1 \cdot 2 \cdot \cdots \cdot 99 \cdot 100</math>. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
====Properties==== | ====Properties==== | ||
| Line 205: | Line 168: | ||
:(''x'' · ''y'')''z'' = ''x''(''y'' · ''z''). | :(''x'' · ''y'')''z'' = ''x''(''y'' · ''z''). | ||
| − | :Note | + | :Note: the parentheses mean that the operations inside the parentheses must be done before anything outside the parentheses is done. |
* Multiplication also has what is called a '''[[distributive|distributive property]]''' with respect to the addition, | * Multiplication also has what is called a '''[[distributive|distributive property]]''' with respect to the addition, | ||
| Line 265: | Line 228: | ||
* Every number ''x'', except zero, has a '''[[multiplicative inverse]]''', 1/''x'', such that ''x'' × 1/''x'' = 1. | * Every number ''x'', except zero, has a '''[[multiplicative inverse]]''', 1/''x'', such that ''x'' × 1/''x'' = 1. | ||
| − | * Multiplication by a positive number preserves [[Order theory|order]]: if ''a'' > 0, then if ''b'' > ''c'' then ''ab'' > ''ac''. | + | * Multiplication by a positive number preserves [[Order theory|order]]: if ''a'' > 0, then if ''b'' > ''c'' then ''ab'' > ''ac''. Multiplication by a negative number reverses order: if ''a'' < 0, then if ''b'' > ''c'' then ''ab'' < ''ac''. |
| − | |||
| − | |||
==Division (÷ or /)== | ==Division (÷ or /)== | ||
| Line 399: | Line 360: | ||
==See also== | ==See also== | ||
| + | |||
*[[Addition of natural numbers]] | *[[Addition of natural numbers]] | ||
*[[Additive inverse]] | *[[Additive inverse]] | ||
| Line 407: | Line 369: | ||
*[[Finite field arithmetic]] | *[[Finite field arithmetic]] | ||
*[[Number line]] | *[[Number line]] | ||
| − | |||
*[[Arithmetic coding]] | *[[Arithmetic coding]] | ||
*[[Arithmetic mean]] | *[[Arithmetic mean]] | ||
| Line 418: | Line 379: | ||
==References== | ==References== | ||
| + | ;History | ||
| + | *{{cite book | author=Bunt, Jones, and Bedient | title=The historical roots of elementary mathematics | publisher=Prentice-Hall | year=1976 | id=ISBN 0-13-389015-5}} | ||
| + | *{{cite book | first=José | last=Ferreirós | title=Labyrinth of thought: A history of set theory and its role in modern mathematics | publisher=Birkhäuser | year=1999 | id=ISBN 0-8176-5749-5}} | ||
| + | *{{cite book | first=Robert | last=Kaplan | title=The nothing that is: A natural history of zero| publisher=Oxford UP | year=2000 | id=ISBN 0-19-512842-7}} | ||
| + | *{{cite book | first=Steven | last=Schwartzman | title=The words of mathematics: An etymological dictionary of mathematical terms used in English | publisher=[[Mathematical Association of America|MAA]] | year=1994 | id=ISBN 0-88385-511-9}} | ||
| + | *{{cite book | first=Michael | last=Williams | title=A history of computing technology | publisher=Prentice-Hall | year=1985 | id=ISBN 0-13-389917-9}} | ||
* Cunnington, Susan. The story of arithmetic, a short history of its origin and development. Swan Sonnenschein, London, 1904. | * Cunnington, Susan. The story of arithmetic, a short history of its origin and development. Swan Sonnenschein, London, 1904. | ||
* Dickson, Leonard Eugene. History of the theory of numbers. Three volumes. Reprints: Carnegie Institute of Washington, Washington, 1932. Chelsea, New York, 1952, 1966. | * Dickson, Leonard Eugene. History of the theory of numbers. Three volumes. Reprints: Carnegie Institute of Washington, Washington, 1932. Chelsea, New York, 1952, 1966. | ||
* Fine, Henry Burchard (1858-1928). The number system of algebra treated theoretically and historically. Leach, Shewell & Sanborn, Boston, 1891. | * Fine, Henry Burchard (1858-1928). The number system of algebra treated theoretically and historically. Leach, Shewell & Sanborn, Boston, 1891. | ||
| − | * [[Louis Charles Karpinski|Karpinski, Louis Charles]] | + | * [[Louis Charles Karpinski|Karpinski, Louis Charles]]*. The history of arithmetic. Rand McNally, Chicago, 1925. Reprint: Russell & Russell, New York, 1965. |
* Ore, Øystein. Number theory and its history. McGraw-Hill, New York, 1948. | * Ore, Øystein. Number theory and its history. McGraw-Hill, New York, 1948. | ||
* Weil, Andre. Number theory: an approach through history. Birkhauser, Boston, 1984. Reviewed: Math. Rev. 85c:01004. | * Weil, Andre. Number theory: an approach through history. Birkhauser, Boston, 1984. Reviewed: Math. Rev. 85c:01004. | ||
| + | |||
| + | ;Elementary mathematics | ||
| + | *{{cite book | author=Davison, Landau, McCracken, and Thompson | title=Mathematics: Explorations & Applications | edition = TE | publisher=Prentice Hall | year=1999 | id=ISBN 0-13-435817-1}} | ||
| + | *{{cite book | author=F. Sparks and C. Rees | title=A survey of basic mathematics | publisher=McGraw-Hill | year=1979 | id=ISBN 0-07-059902-5}} | ||
| + | |||
| + | ;Education | ||
| + | *{{cite book | first=Edward | last=Begle | title=The mathematics of the elementary school | publisher=McGraw-Hill | year=1975 | id=ISBN 0-07-004325-6}} | ||
| + | *[http://www.cde.ca.gov/be/st/ss/mthmain.asp California State Board of Education mathematics content standards] Adopted December 1997, accessed December 2005. | ||
| + | *{{cite book | author=D. Devine, J. Olson, and M. Olson | title=Elementary mathematics for teachers | edition=2e | publisher=[[John Wiley & Sons|Wiley]] | year=1991 | id=ISBN 0-471-85947-8}} | ||
| + | *{{cite book | author=National Research Council | title=Adding it up: Helping children learn mathematics | publisher=[[United States National Academies|National Academy Press]] | year=2001 | id=ISBN 0-309-06995-5 | authorlink=United States National Research Council | url=http://www.nap.edu/books/0309069955/html/index.html}} | ||
| + | *{{cite book | first=John | last=Van de Walle | title=Elementary and middle school mathematics: Teaching developmentally | edition=5e | publisher=Pearson | year=2004 | id=ISBN 0-205-38689-X}} | ||
| + | |||
| + | ;Cognitive science | ||
| + | *{{cite conference | author=Baroody and Tiilikainen | title=Two perspectives on addition development | booktitle=The development of arithmetic concepts and skills | year=2003 | pages=75 | id=ISBN 0-8058-3155-X}} | ||
| + | *{{cite book | author=Fosnot and Dolk | title=Young mathematicians at work: Constructing number sense, addition, and subtraction | publisher=Heinemann | year=2001 | id=ISBN 0-325-00353-X}} | ||
| + | *{{cite conference | first=J. Fred | last = Weaver | title=Interpretations of number operations and symbolic representations of addition and subtraction | booktitle=Addition and subtraction: A cognitive perspective | year=1982 | pages=60 | id=ISBN 0-89859-171-6}} | ||
| + | *{{cite conference | first=Karen | last = Wynn | title=Numerical competence in infants | booktitle=The development of mathematical skills | year=1998 | pages=3 | id=ISBN 0-86377-816-X}} | ||
| + | |||
| + | ;Mathematical exposition | ||
| + | *{{cite web | author=Bogomolny, Alexander | year=1996| title=Addition | work=Interactive Mathematics Miscellany and Puzzles (cut-the-knot.org) | url=http://www.cut-the-knot.org/do_you_know/addition.shtml | accessdate=February 3 | accessyear=2006}} | ||
| + | *{{cite book | first=William | last=Dunham | title=The mathematical universe | publisher=Wiley | year=1994 | id=ISBN 0-471-53656-3}} | ||
| + | *{{cite book | first=Paul | last=Johnson | title=From sticks and stones: Personal adventures in mathematics | publisher=Science Research Associates | year=1975 | id=ISBN 0-574-19115-1}} | ||
| + | *{{cite book | first = Carl | last = Linderholm | year = 1971 | title = Mathematics made difficult | publisher = Wolfe | id = ISBN 0-7234-0415-1}} | ||
| + | *{{cite book | first=Frank | last=Smith | title=The glass wall: Why mathematics can seem difficult | publisher=Teachers College Press | year=2002 | id=ISBN 0-8077-4242-2}} | ||
| + | *{{cite book | first=Karl | last=Smith | title=The nature of modern mathematics | edition=3e | publisher=Wadsworth | year=1980 | id=ISBN 0-8185-0352-1}} | ||
| + | |||
| + | ;Advanced mathematics | ||
| + | *{{cite book | first=George | last=Bergman | title=An invitation to general algebra and universal constructions | edition=2.3e | publisher=General Printing | year=2005 | id=ISBN 0-9655211-4-1 | url=http://math.berkeley.edu/~gbergman/245/index.html}} | ||
| + | *{{cite book | first=Claude | last=Burrill | title=Foundations of real numbers | publisher=McGraw-Hill | year=1967 | id={{LCC|QA248.B95}}}} | ||
| + | *{{cite book | author=D. Dummit and R. Foote | title=Abstract algebra | edition=2e | publisher=Wiley | year=1999 | id=ISBN 0-471-36857-1}} | ||
| + | *{{cite book | first=Herbert | last=Enderton | title=Elements of set theory | publisher=[[Academic Press]] | year=1977 | id=ISBN 0-12-238440-7}} | ||
| + | *{{cite book | first=John | last=Lee | title=Introduction to smooth manifolds | publisher=Springer | year=2003 | id=ISBN 0-387-95448-1}} | ||
| + | *{{cite book | first=John | last=Martin | title=Introduction to languages and the theory of computation | publisher=McGraw-Hill | edition=3e | year=2003 | id=ISBN 0-07-232200-4}} | ||
| + | *{{cite book | first=Walter | last=Rudin | title=Principles of mathematical analysis | edition=3e | publisher=McGraw-Hill | year=1976 | id=ISBN 0-07-054235-X}} | ||
| + | *{{cite book | first=James | last=Stewart | title=Calculus: Early transcendentals | edition=4e | publisher=Brooks/Cole | year=1999 | id=ISBN 0-534-36298-2}} | ||
| + | |||
| + | ;Mathematical research | ||
| + | *{{cite journal | author=Akian, Bapat, and Gaubert | title= Min-plus methods in eigenvalue perturbation theory and generalised Lidskii-Vishik-Ljusternik theorem | journal= INRIA reports | year=2005 | url=http://arxiv.org/abs/math.SP/0402090 }} | ||
| + | *{{cite conference | author=[[John Carlos Baez|J. Baez]]* and J. Dolan | title=From Finite Sets to Feynman Diagrams | booktitle=Mathematics Unlimited— 2001 and Beyond | year=2001 | pages=29 | url=http://arxiv.org/abs/math.QA/0004133 | id=ISBN 3-540-66913-2}} | ||
| + | *Litvinov, Maslov, and Sobolevskii (1999). [http://arxiv.org/abs/math.SC/9911126 Idempotent mathematics and interval analysis]. ''[http://www.springerlink.com/openurl.asp?genre=article&eissn=1573-1340&volume=7&issue=5&spage=353 Reliable Computing]'', Kluwer. | ||
| + | *{{cite journal | first=Jean-Louis | last=Loday | title= Arithmetree | journal= J. of Algebra | year=2002 | url=http://arxiv.org/abs/math/0112034 }} | ||
| + | *{{cite journal | author=Mikhalkin, Grigory | title= Tropical Geometry and its applications | journal=To appear at the Madrid [[International Congress of Mathematicians|ICM]] | year=2006 | url=http://arxiv.org/abs/math.AG/0601041 }} | ||
| + | *Viro, Oleg (2000). [http://arxiv.org/abs/math/0005163 Dequantization of real algebraic geometry on logarithmic paper]. ([http://www.math.uu.se/~oleg/dequant/dequantH1.html HTML]) Plenary talk at 3rd [[European Mathematical Society|ECM]], Barcelona. | ||
| + | |||
| + | ;Computing | ||
| + | *{{cite book | author=M. Flynn and S. Oberman | title=Advanced computer arithmetic design | publisher=Wiley | year=2001 | id=ISBN 0-471-41209-0}} | ||
| + | *{{cite book | author=P. Horowitz and W. Hill | title=The art of electronics | edition=2e | publisher=Cambridge UP | year=2001 | id=ISBN 0-521-37095-7}} | ||
| + | *{{cite book | first=Albert | last=Jackson | title=Analog computation | publisher=McGraw-Hill | year=1960 | id={{LCC|QA76.4|J3}}}} | ||
| + | *{{cite book | author=T. Truitt and A. Rogers | title=Basics of analog computers | publisher=John F. Rider | year=1960 | id={{LCC|QA76.4|T7}}}} | ||
==External links== | ==External links== | ||
Revision as of 16:59, 12 December 2006
Arithmetic or arithmetics (from the Greek word αριθμός, meaning "number") is the oldest and most elementary branch of mathematics, used by almost everyone, for tasks ranging from simple daily counting to advanced science and business calculations. In common usage, the word refers to a branch of (or the forerunner of) mathematics which records elementary properties of certain operations on numbers. Professional mathematicians sometimes use the term higher arithmetic[1] as a synonym for number theory, but this should not be confused with elementary arithmetic.
The traditional arithmetic operations are addition, subtraction, multiplication, and division, although more advanced operations (such as manipulations of percentages, square root, exponentiation, and logarithmic functions) are also sometimes included in this subject. Any set of objects upon which all four operations of arithmetic can be performed (except division by zero), and wherein these four operations obey the usual laws, is called a field.
History
Our knowledge of the prehistory of arithmetic is limited by a small number of artifacts indicating a clear conception of addition and subtraction, the best-known being the Ishango Bone from Africa, dating from 18,000 B.C.E.
It is clear that the Babylonians had solid knowledge of almost all aspects of elementary arithmetic circa 1850 B.C.E., historians can only infer the methods utilized to generate the arithmetical results (see Plimpton 322). Likewise, a definitive algorithm for multiplication and the use of unit fractions can be found in the Rhind Mathematical Papyrus dating from Ancient Egypt circa 1650 B.C.E.
In the Pythagorean school, in the second half of the sixth century B.C.E., arithmetic was considered one of the four quantitative or mathematical sciences (Mathemata). These were carried over in mediæval universities as the Quadrivium which, together with the Trivium of grammar, rhetoric and dialectic, constituted the septem liberales artes (seven liberal arts).
Modern algorithms for arithmetic (both for hand and electronic computation) were made possible by the introduction of Arabic numerals and decimal place notation for numbers. Although it is now considered elementary, its simplicity is the culmination of thousands of years of mathematical development. By contrast, the ancient mathematician Archimedes devoted an entire work, The Sand Reckoner, to devising a notation for a certain large integer. The flourishing of algebra in the medieval Islamic world and in Renaissance Europe was an outgrowth of the enormous simplification of computation through decimal notation.
Decimal arithmetic
Decimal notation constructs all real numbers from the basic digits, the first ten non-negative integers 0,1,2,...,9. A decimal numeral consists of a sequence of these basic digits, with the "denomination" of each digit depending on its position with respect to the decimal point: for example, 507.36 denotes 5 hundreds (102), plus 0 tens (101), plus 7 units (100), plus 3 tenths (10-1) plus 6 hundredths (10-2). An essential part of this notation (and a major stumbling block in achieving it) was conceiving of 0 as a number comparable to the other basic digits.
Algorism comprises all of the rules of performing arithmetic computations using a decimal system for representing numbers in which numbers written using ten symbols having the values 0 through 9 are combined using a place-value system (positional notation), where each symbol has ten times the weight of the one to its right. This notation allows the addition of arbitrary numbers by adding the digits in each place, which is accomplished with a 10 x 10 addition table. (A sum of digits which exceeds 9 must have its 10-digit carried to the next place leftward.) One can make a similar algorithm for multiplying arbitrary numbers because the set of denominations {...,102,10,1,10-1,...} is closed under multiplication. Subtraction and division are achieved by similar, though more complicated algorithms.
Addition (+)

Addition is the basic operation of arithmetic. In its simplest form, addition combines two numbers, the addends or terms, into a single number, the sum.
The result of adding two quantities a and b is their sum, a + b. It is sometimes phrased as "a more than b," or "b more than a." For example, 3 + 2 = 5, since 5 is 2 more than 3.
Adding more than two numbers can be viewed as repeated addition; this procedure is known as summation and includes ways to add infinitely many numbers in an infinite series; repeated addition of the number one is the most basic form of counting.
Addition also models many related processes, including joining two collections of objects, repeated incrementation, moving a point across the number line, and representing two successive translations as one.
Notation and terminology
Addition is written using the plus sign "+" between the terms; that is, in infix notation. The result is expressed with an equals sign. For example,
- 1 + 1 = 2
- 2 + 2 = 4
- 5 + 4 + 2 = 11 (see "associativity" below)
- 3 + 3 + 3 + 3 = 12 (see "multiplication" below)
There are also situations where addition is "understood" even though no symbol appears:
- A column of numbers, with the last number in the column underlined, usually indicates that the numbers in the column are to be added, with the sum written below the underlined number.
- A whole number followed immediately by a fraction indicates the sum of the two, called a mixed number.[3] For example,
31⁄2 = 3 + 1⁄2 = 3.5.
This notation can cause confusion, since in most other contexts, juxtaposition denotes multiplication instead.
The numbers or the objects to be added are generally called the "terms", the "addends", or the "summands"; this terminology carries over to the summation of multiple terms. This is to be distinguished from factors, which are multiplied. Some authors call the first addend the augend. In fact, during the Renaissance, many authors did not consider the first addend an "addend" at all. Today, due to the symmetry of addition, "augend" is rarely used, and both terms are generally called addends.[4]
Properties
Addition is commutative and associative so the order in which the terms are added does not matter. <<Please define the terms "commutative" and "associative" here. You may consult articles by these names in Wikipedia.>> The identity element of addition (the additive identity) is 0, that is, adding zero to any number will yield that same number. Also, the inverse element of addition (the additive inverse) is the opposite of any number, that is, adding the opposite of any number to the number itself will yield the additive identity, 0. For example, the opposite of 7 is (-7), so 7 + (-7) = 0.
Subtraction (−)
Subtraction is essentially the opposite of addition. It is denoted by a minus sign "−" in infix notation.
The traditional names for the parts of the formula
- c − b = a
are minuend (c) − subtrahend (b) = difference (a). The words "minuend" and "subtrahend" are virtually absent from modern usage; Linderholm charges "This terminology is of no use whatsoever."[5] However, "difference" is very common.
If the minuend is larger than the subtrahend, the difference will be positive; if the minuend is smaller than the subtrahend, the difference will be negative; and if they are equal, the difference will be zero.
Subtraction is used to model several closely related processes. For example:
- From a given collection, take away (subtract) a given number of objects.
- Combine a given measurement with an opposite measurement, such as a movement right followed by a movement left, or a deposit and a withdrawal.
- Compare two objects to find their difference. For example, the difference between $800 and $600 is $800 − $600 = $200.
Properties
Subtraction is neither commutative nor associative. For that reason, it is often helpful to look at subtraction as addition of the minuend and the opposite of the subtrahend, that is a − b = a + (−b). When written as a sum, all the properties of addition hold.
In mathematics, it is often useful to view or even define subtraction as a kind of addition, the addition of the opposite. We can view 7 − 3 = 4 as the sum of two terms: seven and negative three. This perspective allows us to apply to subtraction all of the familiar rules and nomenclature of addition. Although subtraction is not associative or commutative, the addition of signed numbers is both.
Basic subtraction: integers
Imagine a line segment of length b with the left end labeled a and the right end labeled c. Starting from a, it takes b steps to the right to reach c. This movement to the right is modeled mathematically by addition:
- a + b = c.
From c, it takes b steps to the left to get back to a. This movement to the left is modeled by subtraction:
- c − b = a.
Now, imagine a line segment labelled with the numbers 1, 2, and 3. From position 3, it takes no steps to the left to stay at 3, so 3 − 0 = 3. It takes 2 steps to the left to get to position 1, so 3 − 2 = 1. This picture is inadequate to describe what would happen after going 3 steps to the left of position 3. To represent such an operation, the line must be extended.
To subtract arbitrary natural numbers, one begins with a line containing every natural number (0, 1, 2, 3, 4, ...). From 3, it takes 3 steps to the left to get to 0, so 3 − 3 = 0. But 3 − 4 is still invalid since it again leaves the line. The natural numbers are not a useful context for subtraction.
The solution is to consider the integer number line (…, −3, −2, −1, 0, 1, 2, 3, …). From 3, it takes 4 steps to the left to get to −1, so
- 3 − 4 = −1.
Multiplication (× or ·)
Multiplication is in essence repeated addition, or the sum of a list of identical numbers. Multiplication finds the product of two numbers, the multiplier and the multiplicand, sometimes both just called factors.
Multiplication, as it is really repeated addition, is commutative and associative; further it is distributive over addition and subtraction. The multiplicative identity is 1, that is, multiplying any number by 1 will yield that same number. Also, the multiplicative inverse is the reciprocal of any number, that is, multiplying the reciprocal of any number by the number itself will yield the multiplicative identity, 1.
77777777777
In mathematics, multiplication is an elementary arithmetic operation. When one of the numbers is a whole number, multiplication is the repeated sum of the other number.
For example, 7 × 4 is the same as 7 + 7 + 7 + 7.
Fractions are multiplied by separately multiplying their denominators and numerators: a/b × c/d = (ac)/(bd). For example, 2/3 × 3/4 = (2×3)/(3×4) = 6/12 = 1/2.
Multiplication can be defined for real and complex numbers, polynomials, matrices and other mathematical quantities as well. The inverse of multiplication is division.
Computation
The standard methods for multiplying numbers using pencil and paper require a multiplication table of memorized or consulted products of small numbers (typically any two numbers from 0 to 9), however one method, the peasant multiplication algorithm, does not.
Multiplying numbers to more than a couple of decimal places by hand is tedious and error prone. Common logarithms were invented to simplify such calculations. The slide rule allowed numbers to be quickly multiplied to about three places of accuracy. Beginning in the early twentieth century, mechanical calculators automated multiplication of up to 10 digit numbers. Modern electronic computers and calculators have greatly reduced the need for multiplication by hand.
Terminology
The two numbers being multiplied are formally called the multiplicand and the multiplier, respectively. (Some write the multiplier first, and say that 7 × 4 stands for 4 + 4 + 4 + 4 + 4 + 4 + 4, but this usage is less common.) The difference was important in Roman numerals and similar systems where multiplication is transformation of symbols and their addition. For example, to multiply VII by XV one changes the VII to LXX (multiplying VII by X) plus XXV (V times V) plus X (II times V), but to multiply XV by VII one changes XV into LXXV (XV times V) plus XV plus XV (each XV times I).
Because of the commutative property of multiplication, there is generally no need to distinguish between the two numbers so they are more commonly referred to as the factors. The result of the multiplication is referred to as the product.
Notation
Multiplication can be denoted in several equivalent ways. All of the following mean, "5 multiplied by 2":
- 5×2
- 5·2
- (5)2, 5(2), (5)(2), 5[2], [5]2, [5][2]
- 5*2
- 5.2
The asterisk (*) is often used on computers because it is a symbol on every keyboard, but it is rarely used when writing math by hand. This usage originated in the FORTRAN programming language. Frequently, multiplication is implied by Juxtaposition rather than shown in a notation. This is standard in algebra, taking forms like
- 5x and xy
This notation is potentially confusing if variables are permitted to have names longer than one letter, as in computer programming languages. The notation is not used with numbers alone: 52 never means 5 × 2.
If the terms are not written out individually, then the product may be written with an ellipsis to mark out the missing terms, as with other series operations (like sums). Thus, the product of all the natural numbers from 1 to 100 can be written . This can also be written with the ellipsis vertically placed in the middle of the line, as .
Properties
For integers, fractions, real and complex numbers, multiplication has certain properties:
- the order in which two numbers are multiplied does not matter. This is called the commutative property,
- x · y = y · x.
- The associative property means that for any three numbers x, y, and z,
- (x · y)z = x(y · z).
- Note: the parentheses mean that the operations inside the parentheses must be done before anything outside the parentheses is done.
- Multiplication also has what is called a distributive property with respect to the addition,
- x(y + z) = xy + xz.
- Also of interest is that any number times 1 is equal to itself, thus,
- 1 · x = x.
- and this is called the identity property. In this regard the number 1 is known as the multiplicative identity.
- The sum of zero numbers is zero.
- This fact is directly received by means of the distributive property:
- m · 0 = (m · 0) + m − m = (m · 0) + (m · 1) − m = m · (0 + 1) − m = (m · 1) − m = m − m = 0.
- So,
- m · 0 = 0
- no matter what m is (as long as it is finite).
- Multiplication with negative numbers also requires a little thought. First consider negative one (-1). For any positive integer m:
- (−1)m = (−1) + (−1) +...+ (−1) = −m
- This is an interesting fact that shows that any negative number is just negative one multiplied by a positive number. So multiplication with any integers can be represented by multiplication of whole numbers and (−1)'s.
- All that remains is to explicitly define (−1)(−1):
- (−1)(−1) = −(−1) = 1
- However, from a formal viewpoint, multiplication between two negative numbers is (again) directly received by means of the distributive property, e.g:
| (−1) × (−1) | |
| = (−1) × (−1) + (−2) + 2 | |
| = (−1) × (−1) + (−1) × 2 + 2 | |
| = (−1) × (−1 + 2) + 2 | |
| = (−1) × 1 + 2 | |
| = (−1) + 2 | |
| = 1 |
- Every number x, except zero, has a multiplicative inverse, 1/x, such that x × 1/x = 1.
- Multiplication by a positive number preserves order: if a > 0, then if b > c then ab > ac. Multiplication by a negative number reverses order: if a < 0, then if b > c then ab < ac.
Division (÷ or /)
Division is essentially the opposite of multiplication. Division finds the quotient of two numbers, the dividend divided by the divisor. Any dividend divided by zero is undefined. For positive numbers, if the dividend is larger than the divisor, the quotient will be greater than one, otherwise it will be less than one (a similar rule applies for negative numbers and negative one). The quotient multiplied by the divisor always yields the dividend.
Division is neither commutative nor associative. As it is helpful to look at subtraction as addition, it is helpful to look at division as multiplication of the dividend times the reciprocal of the divisor, that is a ÷ b = a × 1⁄b. When written as a product, it will obey all the properties of multiplication.
7777777777 In mathematics, especially in elementary arithmetic, division is an arithmetic operation which is the inverse of multiplication.
Specifically, if c times b equals a, written:
where b is not zero, then a divided by b equals c, written:
For instance,
since
- .
In the above expression, a is called the dividend, b the divisor and c the quotient.
Division by zero (i.e. where the divisor is zero) is usually not defined.
Notation
Division is most often shown by placing the dividend over the divisor with a horizontal line, also called a vinculum, between them. For example, a divided by b is written
This can be read out loud as "a divided by b" or "a over b". A way to express division all on one line is to write the dividend, then a slash, then the divisor, like this:
This is the usual way to specify division in most computer programming languages since it can easily be typed as a simple sequence of characters.
A typographical variation which is halfway between these two forms uses a slash but elevates the dividend, and lowers the divisor:
- a⁄b .
Any of these forms can be used to display a fraction. A fraction is a division expression where both dividend and divisor are integers (although typically called the numerator and denominator), and there is no implication that the division needs to be evaluated further.
A less common way to show division is to use the obelus (or division sign) in this manner:
This form is infrequent except in elementary arithmetic. The obelus is also used alone to represent the division operation itself, as for instance as a label on a key of a calculator.
In some non-English-speaking cultures, "a divided by b" is written a : b. However, in English usage the colon is restricted to expressing the related concept of ratios (then "a is to b").
Examples
Addition table
|
Multiplication table
|
Number theory
The term arithmetic is also used to refer to number theory. This includes the properties of integers related to primality, divisibility, and the solution of equations by integers, as well as modern research which is an outgrowth of this study. It is in this context that one runs across the fundamental theorem of arithmetic and arithmetic functions. A Course in Arithmetic by Serre reflects this usage, as do such phrases as first order arithmetic or arithmetical algebraic geometry. Number theory is also referred to as 'the higher arithmetic', as in the title of H. Davenport's book on the subject.
Arithmetic in education
Primary education in mathematics often places a strong focus on algorithms for the arithmetic of natural numbers, integers, rational numbers (vulgar fractions), and real numbers (using the decimal place-value system). This study is sometimes known as algorism.
The difficulty and unmotivated appearance of these algorithms has long led educators to question this curriculum, advocating the early teaching of more central and intuitive mathematical ideas. One notable movement in this direction was the New Math of the 1960s and '70s, which attempted to teach arithmetic in the spirit of axiomatic development from set theory, an echo of the prevailing trend in higher mathematics [6].
Since the introduction of the electronic calculator, which can perform the algorithms far more efficiently than humans, an influential school of educators has argued that mechanical mastery of the standard arithmetic algorithms is no longer necessary. In their view, the first years of school mathematics could be more profitably spent on understanding higher-level ideas about what numbers are used for and relationships among number, quantity, measurement, and so on. However, most research mathematicians still consider mastery of the manual algorithms to be a necessary foundation for the study of algebra and computer science. This controversy was central to the "Math Wars" over California's primary school curriculum in the 1990s, and continues today [7].
See also
- Addition of natural numbers
- Additive inverse
- Associativity
- Commutativity
- Distributivity
- Elementary arithmetic
- Finite field arithmetic
- Number line
- Arithmetic coding
- Arithmetic mean
- Arithmetic progression
Footnotes
- ↑ Davenport, Harold (1999). The Higher Arithmetic: An Introduction to the Theory of Numbers (7th ed.). Cambridge, England: Cambridge University Press. ISBN 0-521-63446-6.
- ↑ From Enderton (p.138): "...select two sets K and L with card K = 2 and card L = 3. Sets of fingers are handy; sets of apples are preferred by textbooks."
- ↑ Devine et al p.263
- ↑ Schwartzman p.19
- ↑ Linderholm p.42
- ↑ http://www.mathematicallycorrect.com/glossary.htm
- ↑ http://www.education-world.com/a_curr/curr071.shtml
ReferencesISBN links support NWE through referral fees
- History
- Bunt, Jones, and Bedient (1976). The historical roots of elementary mathematics. Prentice-Hall. ISBN 0-13-389015-5.
- Ferreirós, José (1999). Labyrinth of thought: A history of set theory and its role in modern mathematics. Birkhäuser. ISBN 0-8176-5749-5.
- Kaplan, Robert (2000). The nothing that is: A natural history of zero. Oxford UP. ISBN 0-19-512842-7.
- Schwartzman, Steven (1994). The words of mathematics: An etymological dictionary of mathematical terms used in English. MAA. ISBN 0-88385-511-9.
- Williams, Michael (1985). A history of computing technology. Prentice-Hall. ISBN 0-13-389917-9.
- Cunnington, Susan. The story of arithmetic, a short history of its origin and development. Swan Sonnenschein, London, 1904.
- Dickson, Leonard Eugene. History of the theory of numbers. Three volumes. Reprints: Carnegie Institute of Washington, Washington, 1932. Chelsea, New York, 1952, 1966.
- Fine, Henry Burchard (1858-1928). The number system of algebra treated theoretically and historically. Leach, Shewell & Sanborn, Boston, 1891.
- Karpinski, Louis Charles. The history of arithmetic. Rand McNally, Chicago, 1925. Reprint: Russell & Russell, New York, 1965.
- Ore, Øystein. Number theory and its history. McGraw-Hill, New York, 1948.
- Weil, Andre. Number theory: an approach through history. Birkhauser, Boston, 1984. Reviewed: Math. Rev. 85c:01004.
- Elementary mathematics
- Davison, Landau, McCracken, and Thompson (1999). Mathematics: Explorations & Applications, TE, Prentice Hall. ISBN 0-13-435817-1.
- F. Sparks and C. Rees (1979). A survey of basic mathematics. McGraw-Hill. ISBN 0-07-059902-5.
- Education
- Begle, Edward (1975). The mathematics of the elementary school. McGraw-Hill. ISBN 0-07-004325-6.
- California State Board of Education mathematics content standards Adopted December 1997, accessed December 2005.
- D. Devine, J. Olson, and M. Olson (1991). Elementary mathematics for teachers, 2e, Wiley. ISBN 0-471-85947-8.
- National Research Council (2001). Adding it up: Helping children learn mathematics. National Academy Press. ISBN 0-309-06995-5.
- Van de Walle, John (2004). Elementary and middle school mathematics: Teaching developmentally, 5e, Pearson. ISBN 0-205-38689-X.
- Cognitive science
- Baroody and Tiilikainen (2003). "Two perspectives on addition development". The development of arithmetic concepts and skills, 75. ISBN 0-8058-3155-X.
- Fosnot and Dolk (2001). Young mathematicians at work: Constructing number sense, addition, and subtraction. Heinemann. ISBN 0-325-00353-X.
- Weaver, J. Fred (1982). "Interpretations of number operations and symbolic representations of addition and subtraction". Addition and subtraction: A cognitive perspective, 60. ISBN 0-89859-171-6.
- Wynn, Karen (1998). "Numerical competence in infants". The development of mathematical skills, 3. ISBN 0-86377-816-X.
- Mathematical exposition
- Bogomolny, Alexander (1996). Addition. Interactive Mathematics Miscellany and Puzzles (cut-the-knot.org). Retrieved February 3, 2006.
- Dunham, William (1994). The mathematical universe. Wiley. ISBN 0-471-53656-3.
- Johnson, Paul (1975). From sticks and stones: Personal adventures in mathematics. Science Research Associates. ISBN 0-574-19115-1.
- Linderholm, Carl (1971). Mathematics made difficult. Wolfe. ISBN 0-7234-0415-1.
- Smith, Frank (2002). The glass wall: Why mathematics can seem difficult. Teachers College Press. ISBN 0-8077-4242-2.
- Smith, Karl (1980). The nature of modern mathematics, 3e, Wadsworth. ISBN 0-8185-0352-1.
- Advanced mathematics
- Bergman, George (2005). An invitation to general algebra and universal constructions, 2.3e, General Printing. ISBN 0-9655211-4-1.
- Burrill, Claude (1967). Foundations of real numbers. McGraw-Hill. LCC QA248.B95.
- D. Dummit and R. Foote (1999). Abstract algebra, 2e, Wiley. ISBN 0-471-36857-1.
- Enderton, Herbert (1977). Elements of set theory. Academic Press. ISBN 0-12-238440-7.
- Lee, John (2003). Introduction to smooth manifolds. Springer. ISBN 0-387-95448-1.
- Martin, John (2003). Introduction to languages and the theory of computation, 3e, McGraw-Hill. ISBN 0-07-232200-4.
- Rudin, Walter (1976). Principles of mathematical analysis, 3e, McGraw-Hill. ISBN 0-07-054235-X.
- Stewart, James (1999). Calculus: Early transcendentals, 4e, Brooks/Cole. ISBN 0-534-36298-2.
- Mathematical research
- Akian, Bapat, and Gaubert (2005). Min-plus methods in eigenvalue perturbation theory and generalised Lidskii-Vishik-Ljusternik theorem. INRIA reports.
- J. Baez and J. Dolan (2001). "From Finite Sets to Feynman Diagrams". Mathematics Unlimited— 2001 and Beyond, 29. ISBN 3-540-66913-2.
- Litvinov, Maslov, and Sobolevskii (1999). Idempotent mathematics and interval analysis. Reliable Computing, Kluwer.
- Loday, Jean-Louis (2002). Arithmetree. J. of Algebra.
- Mikhalkin, Grigory (2006). Tropical Geometry and its applications. To appear at the Madrid ICM.
- Viro, Oleg (2000). Dequantization of real algebraic geometry on logarithmic paper. (HTML) Plenary talk at 3rd ECM, Barcelona.
- Computing
- M. Flynn and S. Oberman (2001). Advanced computer arithmetic design. Wiley. ISBN 0-471-41209-0.
- P. Horowitz and W. Hill (2001). The art of electronics, 2e, Cambridge UP. ISBN 0-521-37095-7.
- Jackson, Albert (1960). Analog computation. McGraw-Hill. LCC QA76.4 J3.
- T. Truitt and A. Rogers (1960). Basics of analog computers. John F. Rider. LCC QA76.4 T7.
External links
Credits
New World Encyclopedia writers and editors rewrote and completed the Wikipedia article in accordance with New World Encyclopedia standards. This article abides by terms of the Creative Commons CC-by-sa 3.0 License (CC-by-sa), which may be used and disseminated with proper attribution. Credit is due under the terms of this license that can reference both the New World Encyclopedia contributors and the selfless volunteer contributors of the Wikimedia Foundation. To cite this article click here for a list of acceptable citing formats.The history of earlier contributions by wikipedians is accessible to researchers here:
- Arithmetic history
- Addition history
- Subtraction history
- Multiplication history
- Division_(mathematics) history
The history of this article since it was imported to New World Encyclopedia:
Note: Some restrictions may apply to use of individual images which are separately licensed.